About me and why I created this physics website.

The Physics Of Curling

Source: http://www.flickr.com/photos/popejon2/4366766259


Physics Of Curling – The Curling Stone

Curling stones are thick stone disks made of heavy, polished granite, with a handle attached to the top (shown in the picture below). Curling regulations state that the maximum mass of a curling stone is 20 kilograms. The typical mass of a curling stone lies between 17 and 20 kg. The heavy mass of the stone is what enables it to slide down the length of the rink, without slowing down prematurely.

The maximum allowable circumference of a stone is 36 inches (910 mm). The minimum height of a stone is 4.5 inches (110 mm).



Source: http://en.wikipedia.org/wiki/Curling. Author: http://en.wikipedia.org/wiki/File:Curlingrock.jpg


On the base of the curling stone there is a thin annulus called the running band, which supports the weight of the stone. Thus, the running band is the part of the stone in contact with the ice. It is in the shape of a ring, about 12 cm in diameter. The width of the band is about 5 mm when the stone is new, and as the stone wears the width can increase to 8 mm.

The texture of the band and the sharpness or smoothness of the band's edges influences the friction of the stone with the ice and therefore affects the stone's behaviour as it travels down the ice.

The curler can cause the stone to curve on the ice by giving the stone an initial slow rotation. The rotation of the stone is what causes the stone to curve (curl) on the ice as it slides. The stone's path on the ice may be further influenced by two sweepers with brooms who "sweep" the ice in front of the stone as it slides.

The initial speed of the curling stone on the ice is usually a few meters per second, and the curling stone can make 1-4 full revolutions before coming to rest.


Physics Of Curling – Analysis Of The Curl

The most intriguing question related to the physics of curling is, why does a curling stone curve (curl) on the ice?

Interestingly, curling is the only sport where the trajectory of the projectile can be influenced after release. Players "sweep" the ice directly in front of the curling stone to decrease the friction of the stone with the ice. This in turn affects the degree of curl of the stone as it slides down the length of the rink, towards the target area.

To understand why a curling stone curls we must analyze the physics of curling. The figure below shows a schematic of the curling physics, for a curling stone rotating clockwise.



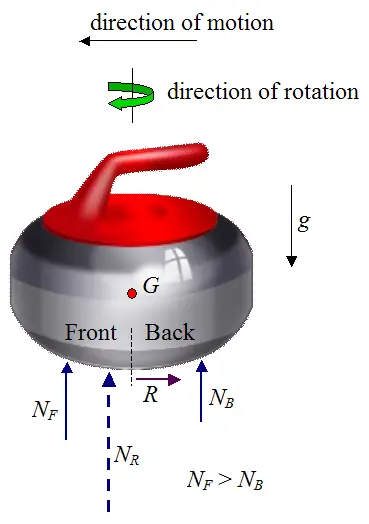
Where:

g is the acceleration due to gravity (equal to 9.8 m/s² on earth)

G is the center of mass of the curling stone, which by symmetry is assumed to lie on the geometric center line of the stone

N_F is the resultant normal force exerted on the bottom front half of the curling stone, due to contact with the ice

N_B is the resultant normal force exerted on the bottom back half of the curling stone, due to contact with the ice

N_R is the resultant normal force exerted on the (entire) bottom of the curling stone, due to contact with the ice. (Note that N_R = N_F + N_B)

R is the resultant component of friction force exerted on the (entire) bottom of the curling stone, that is acting opposite the direction of motion of the stone. This friction force is due to contact with the ice, and acts to slow down the stone as it slides down the ice


Since the curling stone is in rotational equilibrium in the plane of the page, the sum of the moments about G in this plane must sum to zero. This means that the normal force N_R must exert a moment in the opposite direction to the moment caused by R. This is only possible if the line of action of N_R is to the left of G (on the bottom front half of the curling stone). Consequently, most of the normal force is acting on the bottom front half, and N_F > N_B. (Note that we are ignoring three-dimensional effects which are assumed to be negligible).

It is a known fact that contact pressure with ice melts a very thin layer of water on the surface of the ice. This in turn decreases friction. Thus, the friction is inversely proportional to the amount of contact pressure with the ice. So, as a result of N_F > N_B, the friction force exerted on the (bottom) front half of the curling stone (due to contact with the ice) is less than the friction force exerted on the (bottom) back half of the curling stone (due to contact with the ice). The figure below illustrates this.



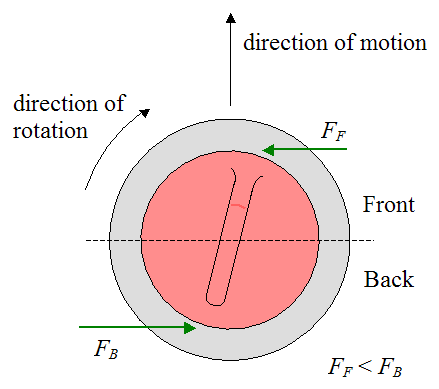
Where:

F_F is the resultant component of friction force exerted on the (bottom) front half of the curling stone, opposing the direction of rotation

F_B is the resultant component of friction force exerted on the (bottom) back half of the curling stone, opposing the direction of rotation

As stated previously, F_F < F_B. As a result, the clockwise rotation of the curling stone causes it to move to the right due to the greater friction force F_B. If the curling stone is rotated counterclockwise then F_F and F_B will be pointing in opposite directions and the stone will instead curl to the left.

This is an interesting result. By imparting spin on the curling stone, friction is induced which steers the stone away from its initial straight line trajectory.

The challenge for curlers is to control the level of curl of the stone, in order to get it where they want in the target area. They do this by controlling the level of friction of the stone with the ice. This aspect of the physics will be discussed next.


Controlling The Curl By Controlling Ice Friction

Controlling ice friction is achieved in basically two ways. The first way is in the form of pregame preparation, in which fine water droplets are sprayed onto the flat ice surface. These droplets freeze into small "pebbles", which the curling stones "ride" on as they slide down the ice. These pebbles reduce friction and enable the stones to travel farther (and curl less) than if the ice is not pebbled. The reason for this is because the pebbles form the contact area with the running band, which concentrates the weight of the stone onto a smaller ice area than if there was only flat (pebble-less) ice. This increases contact pressure which lowers the friction of the stone with the ice. As a result, the stones travel farther, and curl less.

The second way to control ice friction (which is done during a game), is by having other players on the team "sweep" in front of the curling stone. The sweeping action removes any dirt or debris, but more importantly it also temporarily raises the surface temperature of the ice (by a small amount). This reduces the friction between stone and ice, which causes the stone to travel further and curl less.

Different stages of the stone's travel on the ice may require different trajectory adjustments. One way to accomplish this is by adjusting the sweeping intensity and/or strategy, which affects the stone-ice friction, and as a result affects the rate of curl. For example, the sweepers can be strategically placed so that they are opposite each other, with the sweeper closest to the stone having the most influence on the friction between stone and ice. The sweeper closest to the stone has the most influence because the proximity of his sweeping action gives more time for the stone to travel on the swept (lower friction) surface before the ice re-cools and friction is restored to its previous (higher) level. In addition, the side the closest sweeper is on can also have some influence on how much the stone curls.

Interestingly, the curling stone tends to curl more near the end of its travel (during which the stone slows down to a stop). One possible reason for this is because the thin layer of melted water on the contact interface is dragged around to the front of the stone (due to the stone's rotation), especially during the final few feet of travel. This effect causes the friction force F_F to become even smaller relative to F_B. As a result, the rate of curl increases in the final few feet of travel. (ref: What Puts the Curl in a Curling Stone?, Mark Shegelski, Canadian Curling News, March, 2000).

Clearly, the physics of curling can become quite complex when one tries to account for all the factors that come into play.


Temperature And Humidity

To ensure optimal curling conditions, the surface temperature of the ice is maintained at around -5 degrees Celsius. In addition, the humidity of the air in the rink is deliberately kept low in order to prevent condensation of water on the ice, which can adversely affect play conditions.

The temperature of the running band of the curling stone is kept at the same temperature as the ice to ensure proper play. If the running band is warmer than the ice surface, increased molecular bonding will result. This will increase friction with the ice, which will adversely affect play.



Return to The Physics Of Sports page


Return to Real World Physics Problems home page